MRI (Magnetic Resonance Imaging)
 
The human trunk is represented by a dielectric cylinder. The excitation pulse is a sinusoidal wave, and we calculate the impedance through calculation of the amplitude difference in the first two periods. The permeability of filled material inside the cavity is 8257, 9214 and 14800 in three test cases, respectively. The wavelength inside the cavity is much smaller than that in free space. In turn, the cell size is very small to describe the field variation inside the cavity.
 
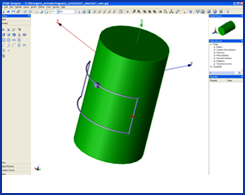
Configuration:Cylinder: er=81, s=0.6S/m
Cylinder radius: 8.5 cm
Cylinder height: 28 cm
Wire: copper
Wire major radius: 10.15 cm
Wire minor radius: 0.15 cm
-----------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
Simulation result for
test case 1: 		Cylinder: (8.875, -0.95, 0)
Dielectric inside capacitor: permittivity = 8257; permeability = 0.999991
R_simulated = 137.1 mOhms
R_measured = 140.0 mOhms
-----------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
Simulation result for
test case 2: 		Cylinder: (9.3, -0.65, 0)
Dielectric inside capacitor: Permittivity = 9214; permeability = 0.999991
R_simulated = 178.3 mOhms
R_measured = 157.0 mOhms
-----------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
Simulation result for
test case 3: 		Cylinder: (9.8, -0.95, 0)
Dielectric inside capacitor: Permittivity = 14800; permeability = 0.999991
R_simulated: 227.2 mOhms
R_measured: 196.5 mOhms

Magnetic Resonance Imaging (MRI)

MRI simulation project configuration

Thin wire loop and cavityCavity structure